Single Asset Risk: Standard Deviation and Coefficient of Variation

The return of any investment has an average, which is also the expected return, but most returns will be different from the average: some will be more and others will be less than the average. The more individual returns deviate from the expected return, the greater the risk and the greater the potential reward. The degree to which all returns for a particular investment or asset deviate from the expected return of the investment is a measure of its risk.

Standard Deviation: Measure of Absolute Risk

If you recorded the returns of a sample population of investors who invested in 5-year Treasury notes (T-notes), you would note that everyone received a constant rate of return that didn’t deviate, since, once bought, T-notes pay a constant rate of interest with no credit risk. On the other hand, if you had recorded the returns of a sample of investors who had invested in small stocks at the same time, you would see a much wider variation in their returns—some would have done much better than the T-note investors, while others would have done worse, and each of their returns would vary over time.

This variability of returns is commensurate with the investment’s risk, and this risk can be quantified by calculating the standard deviation of this variability. Standard deviation, as applied to investment returns, is a quantitative statistical measure of the variation of specific returns to the average of those returns.

Standard Deviation Formula for Investment Returns

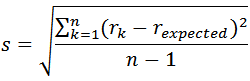s = Standard Deviation rk = Specific Return rexpected = Expected Return n = Number of Returns (sample size).

Coefficient of Variation: Measure of Relative Risk

The greater the standard deviation, the greater the risk of an investment. However, the standard deviation cannot be used to compare investments unless they have the same expected return. For instance, consider the following table.

On the left hand side, you have an investment with an expected return of $5 where each specific return deviates by $1 from the expected return. On the right hand side, the specific returns also deviate by $1, but the expected return is $10. Now because the difference between the expected returns and the specific returns for each sample is 1, the standard deviation is the same, but, nonetheless, the risk is not the same, because $1 is only 10% of $10, but 20% of $5.

The coefficient of variation is a better measure of risk, quantifying the dispersion of an asset’s returns in relation to the expected return, and, thus, the relative risk of the investment. Hence, the coefficient of variation allows the comparison of different investments.

Coefficient of Variation = Standard Deviation / Average Return

In the above case, both samples have the same standard deviation, but have a significant difference in the coefficient of variation. It is obvious that the investment with the smaller return has the greater risk in this case.

So while the standard deviation measures the dispersion of returns, the coefficient of variation measures their relative dispersion.

Example — Calculating the Standard Deviation and Coefficient of Variation

Using the data from Sample 1 in the above table, where the average or expected return = 5, and the formulas for the standard deviation and coefficient of variation and remembering that x^1/2 = √x, we find that:

Microsoft Excel also has a function to calculate the standard deviation, STDEV, using the format STDEV(number 1, number 2, ...), an example calculation is also shown in the above table for Sample 1. You can also select the numbers in the table as the input to the STDEV function. There is no Excel function for the coefficient of variation, but it is simple enough to calculate, knowing the standard deviation.